A Formulation of the Many-Body Expansion (MBE) for Infinite Periodic Systems
This work demonstrates the extension of the MBE for periodic systems and its use to decompose the lattice energies of different ice polymorphs
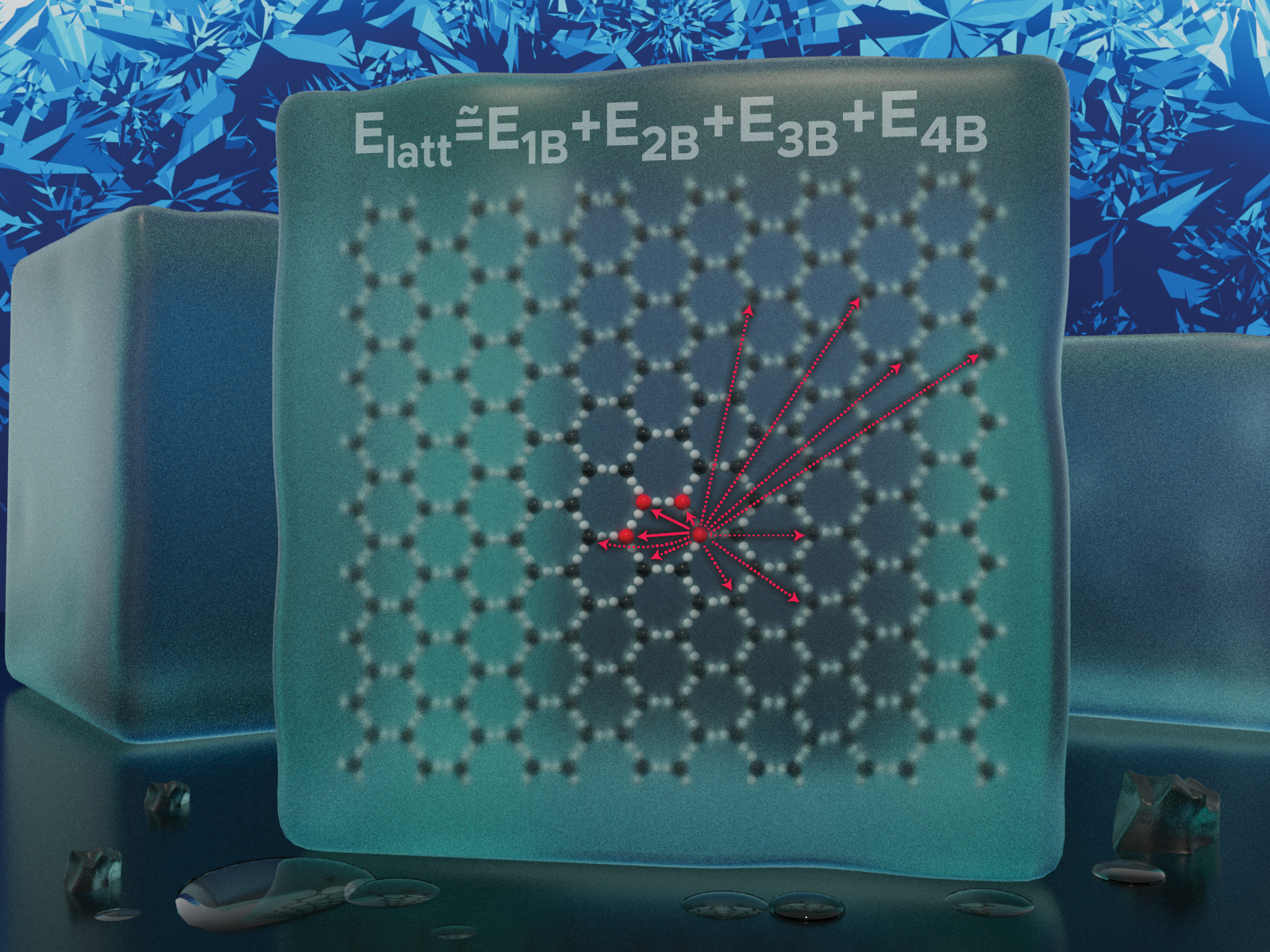
Water molecules can form a variety of lattice structures in ice. The formulation of the many-body expansion introduced here, which includes up to the 4-body term, was applied to seven different ice polymorphs and validated against the lattice energies obtained using the full periodic conditions.
(Illustration by Cortland Johnson | Pacific Northwest National Laboratory)
The Science
The many-body expansion (MBE) is a useful tool for dissecting the components of the intermolecular interactions in large chemical systems. Although the application of the MBE for finite systems is straightforward, its extension to infinite periodic systems—such as those in ice crystals—has not been fully developed before. Periodic boundary conditions that are present in infinite systems introduce complications that are difficult to incorporate into the MBE scheme. In this work, researchers devised a formulation of the MBE for infinite periodic systems and applied it to several ice phases. Their method uses the inherent translational symmetry of the system to define the unique subsystems that are needed in the computation. The researchers then validated this formulation by comparing the lattice energies of seven different ice polymorphs computed via the MBE scheme up to the 4-body term with the results obtained from periodic boundary conditions with Ewald summation as a proof-of-principle.
The Impact
This work showcases the extension of the MBE to infinite periodic systems, opening the possibility to expand the properties of other molecular systems such as carbon compounds (graphite, diamond, graphene, and nanotubes). A comparison of the results from the new MBE formulation with those obtained from periodic boundary conditions with an Ewald summation serves as proof-of-principle of the proposed formulation.
Summary
Researchers devised an extension of the MBE for infinite periodic systems based on the inherent translational symmetry of the system. Beginning with finite gas-phase supercells comprised of replicated unit cells, the scientists formulated a proof-of-principle method showing that their many-body method can: 1) compute unique interactions based on translational symmetry, 2) scale the calculations appropriately, and 3) recover the full n-body terms at a fraction of the computational cost.
The researchers then extended these calculations into infinite periodic systems and validated them against the results attained using periodic boundary conditions with an Ewald summation for seven different polymorphs of ice. The many-body contributions of lattice energy vary significantly among the seven ice phases, ranging from 7 to 24 percent of the total lattice energy. The results indicate that the new MBE formulation can successfully handle the strong long-range and non-additive many-body interactions within the ice lattice. For the first time, the lattice energy of an ice polymorph can be decomposed into the pairwise additive and many-body non-additive components of the respective complex interactions in ice. Ultimately, these results suggest that the MBE can be extended to other molecular properties.
Contact
Sotiris Xantheas
Lab Fellow, Pacific Northwest National Laboratory
Sotiris.Xantheas@pnnl.gov
Funding
This work was supported by the Center for Scalable Predictive Methods for Excitations and Correlated Phenomena (SPEC), which is funded by the Department of Energy, Office of Science, Basic Energy Sciences, Chemical Sciences, Geosciences, and Biosciences Division as part of the Computational Chemical Sciences program at Pacific Northwest National Laboratory.
Published: May 24, 2023
Herman, K. M., and Xantheas, S. S. (2023). A Formulation of the Many-Body Expansion (MBE) for Periodic Systems: Application to Several Ice Phases. The Journal of Physical Chemistry Letters (Vol. 14, Issue 4, pp. 989–999). American Chemical Society (ACS). https://doi.org/10.1021/acs.jpclett.2c03822